inermodz
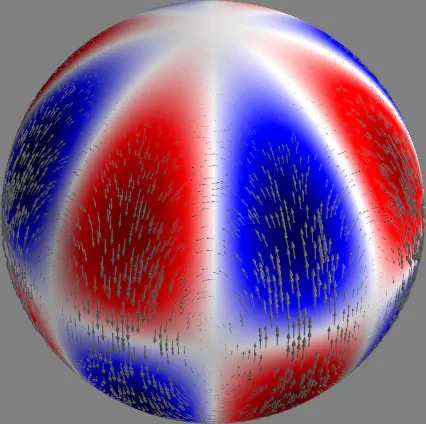 The
$(5,4)$ inertial mode
The
$(5,4)$ inertial modeA fluid rotating with solid body rotation (rotation rate $\Omega$) is stably stratified in angular momentum. It can be shown that a small perturbation to this fluid gives rise to a wave solution that oscillates with frequency $|\omega|\leq2\Omega$. In the absence of boundaries (or far away from them), such a wave propagates as plane waves called “inertial waves”. In the presence of boundaries, the solution must satisfy boundary conditions (for example, impenetrability, $\boldsymbol{u}\cdot\hat{\boldsymbol{n}}=0$) and the solutions are global modes called “inertial modes”. These modes can be computed analytically for some container shapes such as a cylinder and a sphere. This python package allows one to compute the analytical mode frequencies and solutions in a sphere.